A regular quadrilateral is a polygon with four sides that are equal in length and four angles that are equal in measure. This means that all sides of a regular quadrilateral are congruent and all angles are congruent to each other. The internal angles of a regular quadrilateral always add up to 360 degrees. The most common example of a regular quadrilateral is a square.
A square is a type of regular quadrilateral where all sides are equal in length and all angles are equal to 90 degrees. The diagonals of a square are also equal in length and bisect each other at a 90-degree angle. This makes a square a special type of regular quadrilateral as it satisfies all the conditions of a regular quadrilateral.
Another type of regular quadrilateral is a rhombus. A rhombus is a quadrilateral with all sides equal in length, but the angles are not necessarily equal. The opposite angles of a rhombus are equal, but the adjacent angles are not necessarily equal. The diagonals of a rhombus are also perpendicular bisectors of each other.
A regular quadrilateral can also be a parallelogram. A parallelogram is a quadrilateral with opposite sides parallel to each other. The opposite sides of a parallelogram are also equal in length. The angles of a parallelogram are not necessarily equal, but the opposite angles are congruent.
Trapezium is another type of quadrilateral that is not regular sine its sides are of different lengths and angles are not equal. The opposite sides of a trapezium are parallel to each other, but the other two sides are not parallel.
A regular quadrilateral is a polygon with four sides of equal length and four angles of equal measure. A square is the most common example of a regular quadrilateral. Other examples include rhombus and parallelogram. These shapes have unique properties that make them useful in various applications, such as in architecture and engineering. Understanding the properties of regular quadrilaterals is important in geometry and mathematics.
What Shapes Are A Regular Quadrilateral?
A regular quadrilateral is a polygon with four sides, where all sides are equal in length and all angles are congruent. The only shape that qualifies as a regular quadrilateral is a square. Other types of quadrilaterals, such as rectangles, rhombuses, and parallelograms, may have four sides and four angles, but they do not meet the criteria for being a regular quadrilateral. The diagonal of a regular quadrilateral must also bisect each other, which is a characteristic unique to a square. Therefore, a square is the only shape that can be considered a regular quadrilateral.
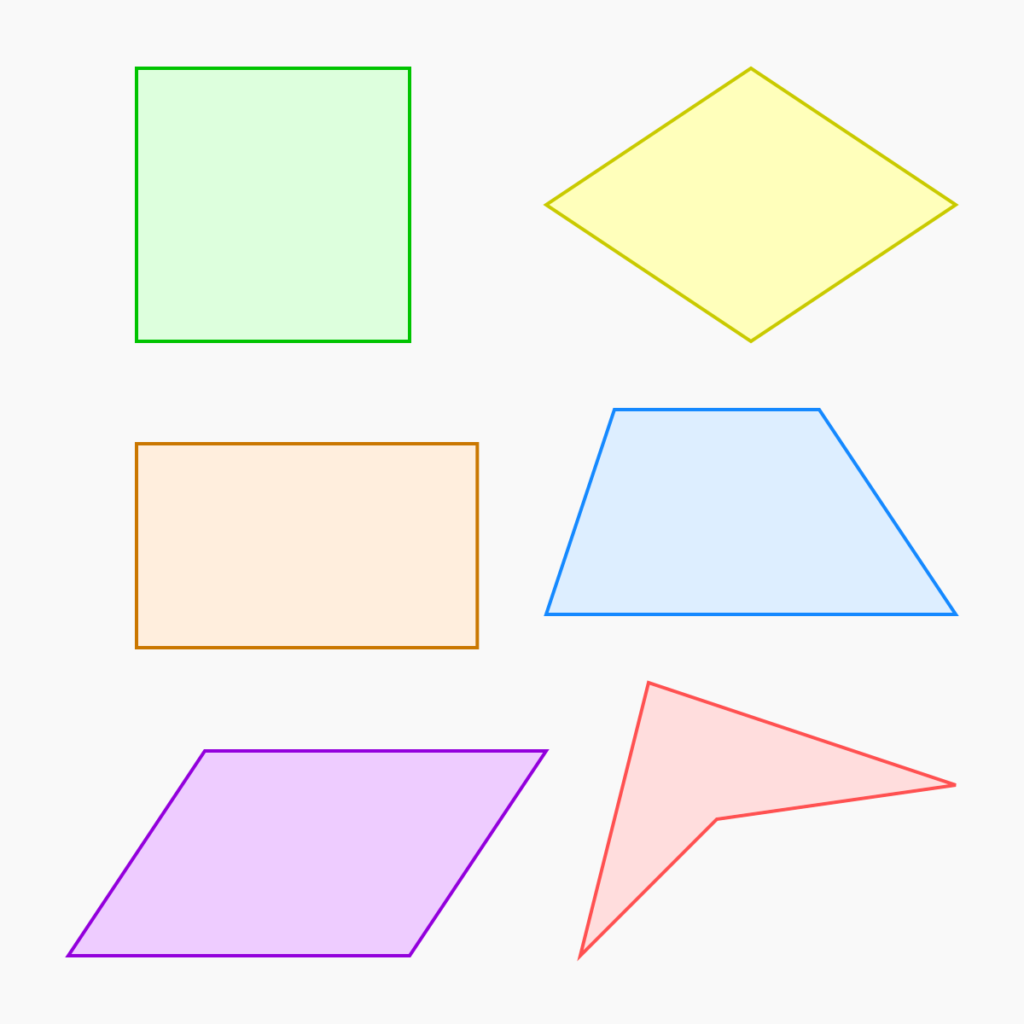
What Are Regular And Irregular Quadrilaterals?
Regular and irregular quadrilaterals are two types of four-sided polygons. A regular quadrilateral, also known as a square, has four sides of equal length. The internal angles of a regular quadrilateral are all 90 degrees. The square is the most common example of a regular quadrilateral.
On the other hand, an irregular quadrilateral has four sides of unequal length. The internal angles of an irregular quadrilateral are not all 90 degrees, and they vary depending on the specific shape. Some examples of irregular quadrilaterals include trapezoids, parallelograms, and kites.
It is important to note that dspite their differences, both regular and irregular quadrilaterals have internal angles that add up to 360 degrees. Additionally, quadrilaterals can be further classified based on their properties, such as whether they are convex or concave.
Regular quadrilaterals have four equal sides and internal angles of 90 degrees, while irregular quadrilaterals have four unequal sides and varying internal angles.
Why Is A Square Called A Regular Quadrilateral?
A square is called a regular quadrilateral because it satisfies two criteria for regularity in quadrilaterals. Firstly, all four sides of a square are equal in length, which means that it is equilateral. Secondly, all four angles of a square are 90 degrees, which means that it is also equiangular. These two conditions of equilateral sides and equiangular corners make the square a regular quadrilateral. a square is a type of regular quadrilateral because it has four equal sides and four equal angles.
Which Quadrilaterals Is Always Regular?
A regular quadrilateral is a polygon with four sides and four angles that are all equal. The only quadrilateral that satisfies this condition is a square. Therefore, a square is always regular. On the other hand, all other quadrilaterals are irregular since they have sides and angles of different lengths and measures. Some examples of irregular quadrilaterals include rectangles, rhombuses, trapezoids, and kites. It is important to note that irregular quadrilaterals can still have some special properties or characteristics that make them useful in geometry and other fields.
Conclusion
A regular quadrilateral is a polygon with four sides of equal length and four angles of equal measure. It is a special type of quadrilateral that has unique properties that distinguish it from other quadrilaterals. The only regular quadrilateral is a square, whch is characterized by its four right angles, diagonals that bisect each other, and four sides of equal length. Regular quadrilaterals are important in geometry as they are used to solve various problems and in many real-life applications such as construction, architecture, and engineering. It is essential for students and learners to understand the properties of regular quadrilaterals to make progress in their studies of geometry. a regular quadrilateral is a fundamental concept in geometry that plays a crucial role in various fields of study.
