Quadratic functions are some of the most important functions in mathematics. They are widely used in physics, engineering, and many oher fields. A quadratic function is a polynomial function of the form f(x) = ax^2 + bx + c, where a, b, and c are constants. In this blog post, we will explore what quadratic functions are, how they work, and whether or not they have asymptotes.
Firstly, let us understand what a quadratic function is. A quadratic function is a second-degree polynomial function, which means that the highest exponent of the variable x is 2. It is a type of function that describes a parabolic curve, which can either be concave up or concave down depending on the sign of the coefficient a. If a is positive, the parabola opens upward, while if a is negative, the parabola opens downward.
Quadratic functions have many important applications in the real world. For example, they are used to model the motion of objects under the influence of gravity or other forces. They are also used to represent the shape of lenses and mirrors in optics, as well as the shape of certain types of curves in geometry.
Now, let us turn our attention to the question of whether or not quadratic functions have asymptotes. The answer is no, quadratic functions do not have asymptotes. Unlike rational functions, which can have vertical or horizontal asymptotes, quadratic functions are smooth and continuous curves that do not have any abrupt changes in direction.
To understand why quadratic functions do not have asymptotes, it is helpful to consider their graph. The graph of a quadratic function is a parabola, which is a smooth curve that does not have any sudden jumps or breaks. As we move along the x-axis, the graph of the function changes smoothly and continuously, without any sharp turns or discontinuities.
Quadratic functions are an important type of function that describes parabolic curves. They are widely used in mathematics, physics, engineering, and many other fields. Unlike rational functions, quadratic functions do not have asymptotes. They are smooth and continuous curves that do not have any abrupt changes in direction. Understanding quadratic functions and their properties is essential for anyone studying mathematics or science, and can lead to a deeper appreciation of the beauty and elegance of mathematics.
Finding Asymptotes of a Quadratic Function
Asymptotes are straight lines that a curve approaches but never touches. Quadratic functions are curves, not lines, and they do not have asymptotes. However, if you are referring to finding the asymptotes of a rational function, which is a function that has a polynomial in the numerator and a polynomial in the denominator, then you can follow the steps outlined in the previous answer.
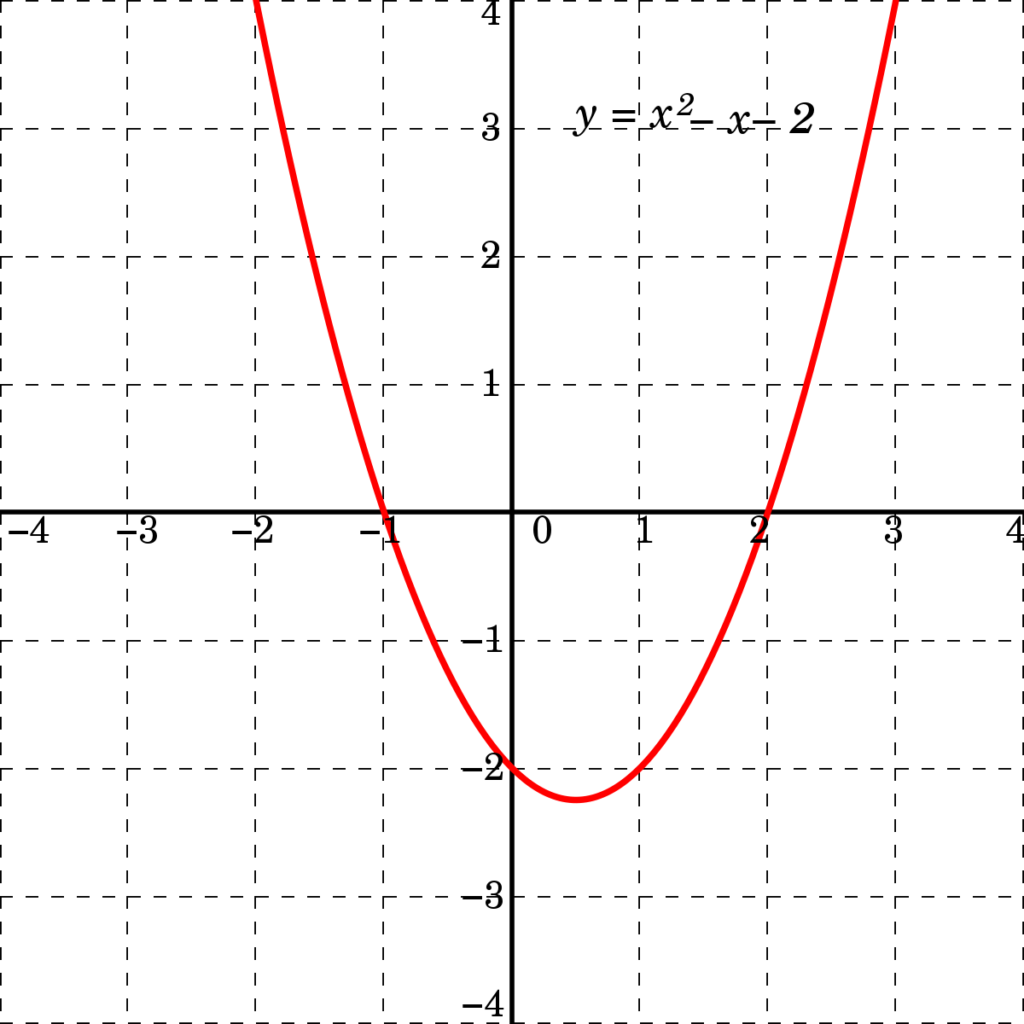
Do All Functions Have Asymptotes?
No, not all functions have asymptotes. Asymptotes are lines that the graph of a function approaches but never touches or crosses. They can be horizontal, vertical, or slanted.
Some functions, such as linear functions, have no asymptotes. They have a constant slope and their graph extends infinitely in both directions.
Other functions, such as quadratic functions, may have horizontal or vertical asymptotes depending on their degree and leading coefficient. For example, a parabola with a vertical axis of symmetry will have a vertical asymptote at the vertex.
However, not all functions have asymptotes. For example, the graph of a constant function is a horizontal line and does not approach any vertical or slanted lines. Similarly, the graph of a circle is a closed curve with no asymptotes.
Rational functions, which are defined as the ratio of two polynomials, may have vertical asymptotes where the denominator is equal to zero. However, not all rational functions have vertical asymptotes. It depends on the factors of the numerator and denominator polynomials.
In summary, whie some functions may have asymptotes, not all functions do. It depends on the shape and behavior of the function’s graph.
Functions Without Asymptotes
Functions that have no asymptotes are typically polynomials. This is because the graphs of polynomials are smooth and continuous, meaning they have no breaks or sharp turns. Additionally, polynomials do not have any vertical asymptotes, which can arise from denominator factors that could be zero in rational algebraic functions.
Other functions that may not have asymptotes include exponential functions, logarithmic functions, and trigonometric functions. However, it is important to note that these functions can have horizontal or slant asymptotes, which occur when the function approaches a certain value as x approaches infinity or negative infinity.
Can Quadratic Functions Have Vertical Asymptotes?
No, a quadratic function cannot have a vertical asymptote. Asymptotes are lines that a function approaches but never touches. A vertical asymptote occurs when the function approaches infinity or negative infinity as the input approaches a specific value, but the function does not cross that value. However, quadratic functions are smooth, continuous curves that do not have any breaks or jumps. They have a fixed degree of curvature and cannot approach infinity in a vertical direction. Therefore, quadratic functions do not have vertical asymptotes.
Function Types with Asymptotes
Many types of functions can have asymptotes. The three most common types of asymptotes are vertical, horizontal, and oblique. Vertical asymptotes occur when the function approaches positive or negative infinity as x approaches a crtain value. Horizontal asymptotes occur when the function approaches a constant value as x approaches positive or negative infinity. Oblique asymptotes occur when the function approaches a straight line as x approaches positive or negative infinity. These types of asymptotes can occur in polynomial, rational, exponential, logarithmic, and trigonometric functions. It is important to note that not all functions have asymptotes, and those that do may have multiple asymptotes of different types.
Source: youtube.com
Can a Function Exist Without Asymptotes?
Yes, it is possible for a function to have no asymptotes. An asymptote is a straight line that a curve approaches but nver touches. In the case of a rational function, if the degree of the numerator is less than or equal to the degree of the denominator, then the function may have one or more horizontal asymptotes or one or more oblique asymptotes. However, if the degree of the numerator is greater than the degree of the denominator, then the function will not have any horizontal asymptotes. In this case, the function may still have oblique asymptotes, but it is also possible for the function to have no asymptotes at all. This means that the curve of the function will not approach any straight line as x approaches positive or negative infinity.
Equation Without Horizontal Asymptote
An equation that has no horizontal asymptote is one in which the degree of the numerator is greater than the degree of the denominator. This is because the numerator grows faster than the denominator as x approaches infinity or negative infinity, so the function does not approach a constant vale as x increases or decreases without bound. Therefore, the graph of such an equation will not have a horizontal line that it approaches as x goes to infinity or negative infinity. Instead, the graph may have oblique or slant asymptotes, or it may have no asymptotes at all. It is important to note that an equation with no horizontal asymptote can still have vertical asymptotes or other types of asymptotes.
Equations with Asymptotes
Equations that have asymptotes are typically rational functions, whch are expressed as a ratio of polynomials. These functions may have horizontal, vertical, or slant asymptotes. A horizontal asymptote is present when the degree of the numerator is less than or equal to the degree of the denominator. In this case, the horizontal asymptote is located at the ratio of the leading coefficients of the numerator and denominator. A vertical asymptote is present when the denominator of the rational function is equal to zero, but the numerator is not. The equation of the vertical asymptote is given by the value that makes the denominator zero. Finally, a slant asymptote is present when the degree of the numerator is greater than the degree of the denominator by one. In this case, the slant asymptote is found by performing polynomial long division, and the equation of the slant asymptote is given by the quotient obtained in the division. In summary, equations that have asymptotes are most commonly rational functions, and the type of asymptote depends on the degree and structure of the numerator and denominator of the function.
Functions Without Asymptotes
Functions are mathematical tools used to describe relationships between variables. Some functions have asymptotes, which are lines that the graph of the function approaches but never touches. However, not all functions have asymptotes.
Functions that do not have asymptotes are usually continuous and well-behaved. This means that they do not have any sudden jumps or breaks in their graphs. Asymptotes arise when there is a discontinuity or singularity in the function, such as a vertical asymptote when the denominator of a rational function equals zero.
Functions that do not have asymptotes may have a horizontal or vertical line that serves as a limit or boundary for the function. However, this line is not an asymptote becuse the function does not approach it in any specific way.
In summary, functions may or may not have asymptotes depending on their behavior and properties. Functions that are continuous and well-behaved typically do not have asymptotes, while functions with discontinuities or singularities often have one or more asymptotes.
Source: en.wikipedia.org
Determining the Presence of Vertical Asymptotes
To determine if a function has any vertical asymptotes, we need to check if the denominator of the fraction in the function can be equal to zero. If we find any value of x that makes the denominator zero, then the function will have a vertical asymptote at that x value.
However, if we solve for the denominator of the fraction and don’t get any real solution for x, then we can conclude that the function does not have any vertical asymptotes. This is beause for a vertical asymptote to exist, the denominator must be zero at some point, and since we didn’t find any real values of x that make the denominator zero, we can say that the function has no vertical asymptotes.
In summary, to determine if a function has any vertical asymptotes, we need to check if the denominator can be equal to zero, and if we don’t find any real solutions for x, then we can conclude that the function has no vertical asymptotes.
Conclusion
In conclusion, quadratic functions are a type of polynomial function that can be written in the form f(x) = ax^2 + bx + c, where a, b, and c are constants. These functions have a parabolic shape and can either open upward or downard depending on the sign of the leading coefficient, a. The vertex of a quadratic function, which represents the minimum or maximum point on the graph, can be found using the formula x = -b/2a. Quadratic functions have many real-world applications, such as in physics, engineering, and finance. It is important to note that quadratic functions do not have vertical or horizontal asymptotes, unlike rational functions. Overall, understanding quadratic functions is essential in higher-level mathematics and in the real world.
