Are ordinal variables continuous? It’s a question many people have asked, and the answer isn’t as simple as you may think. In this blog post, we’ll take a closer look at what ordinal variables are and how they can be both discrete and continuous.
To start off, it helps to understand what an ordinal variable is. An ordinal variable is a type of categorical variable that has values that are ordered in relation to each other. In other words, there is an intrinsic order between the different categories of the variable. For example, in a survey whre respondents are asked to rate their behaviour on a scale from excellent to poor, the responses would be considered an ordinal variable because there is an order between the different responses.
It’s important to note that even though ordinal data can sometimes be numerical (like in the example above), not all mathematical operations can be performed on them. This distinction sets them apart from continuous variables which can be manipulated mathematically in any way.
But here’s where it gets interesting – even thogh ordinal data cannot be manipulated mathematically like continuous data can, it still can act as both discrete and continuous data depending on how it’s used. In other words, even though there is a distinction between continuous and discrete variables, an ordinal variable can still act as either one depending on its purpose or context.
For example, if you were measuring changes in temperature over time (an interval scale) then your data would be considered continuous because you could perform mathematical operations on it like addition or subtraction. However, if you were measuring people’s opinions about something (an ordinal scale) then your data would be considered discrete because you couldn’t perform any mathematical operations on it – instead you could only compare relative rankings between different responses.
So to answer the original question – yes, ordinal variables can act as both discrete and continuous depending on how they are used or interpreted! Understanding this distinction will help make sure that you use your data correctly and get accurate results from your analysis.
Discreteness of Ordinal Variables
Ordinal variables can be either discrete or continuous, depending on how they are used. Discrete ordinal variables denote a value that is distinct from other values in the set, such as ranking items in a survey. Continuous ordinal variables, on the other hand, imply that tere are many possible values between two points on the scale. For example, if a survey asks participants to rate their satisfaction with a product on a 7-point scale from “very dissatisfied” to “very satisfied”, this would be considered a continuous ordinal variable because there are many possible intermediate values between the two endpoints.
Source: codecademy.com
Types of Variables: Ordinal
An ordinal variable is a type of categorical variable, which is used to classify data into groups that have a logical ordering. It is different from a nominal variable in that the values of an ordinal variable are ordered and can be compared to each other. For example, an ordinal variable can represent a scale of ratings such as Excellent, Very Good, Good, Fair, and Poor. The order of the categories implies that Excellent is better than Very Good, and Very Good is better than Good, etc.
Categorizing Ordinal Variables
Ordinal variables are categorical variables. They represent categories or distinct groups that can be ranked in order of importance, but the differences between the categories cannot be precisely determined. Unlike continuous variables, ordinal variables do not have an intrinsic ordering and cannot be measured on an interval or ratio scale. For example, a survey respondent might rate their satisfaction with a product on a scale from 1 (very dissatisfied) to 5 (very satisfied), with no inherent meaning to the difference between each value on the scale.
Are Continuous and Ordinal Variables Different?
No, continuous and ordinal variables are not the same. Continuous variables are numeric values that can take on any value within a given range and can be measured alng a continuum. Examples of continuous variables include height, weight, length, time, and temperature. Ordinal variables are numeric values that represent categories or ranks in an ordered sequence. Examples of ordinal variables include social class rankings, survey responses with predetermined answer choices (i.e., strongly agree, agree, disagree), and Likert scales.
Differences Between Ordinal and Discrete Data
No, ordinal and discrete are not the same. An ordinal variable is a type of categorical variable that has a specific order or ranking associatd with it. This means that the values can be placed in an order from smallest to largest, such as “low,” “medium,” and “high.” On the other hand, a discrete quantitative variable is a numerical value that takes on certain distinct values within an interval. For example, if we are counting the number of cats in a household, the values could be 0, 1, 2, 3, etc., but would never take on fractional or decimal values.
Identifying Continuous Variables
Continuous variables are variables that can take any value within a given range, rathr than having a finite set of distinct values. Examples of continuous variables include age, height, weight, temperature, time, speed, and pressure. Continuous variables can also be measured using units such as seconds, meters per second (speed), or degrees Celsius (temperature). Continuous variables differ from categorical or discrete variables which have a finite set of possible values. For example, gender is a categorical variable with two possible values (male and female).
Describing an Ordinal Variable
An ordinal variable is a type of categorical variable that can take on ordered values. It is used to measure variables that have a natural order, such as rankings or ratings. For example, in a survey, respondents mght be asked to rate their satisfaction with a product from 1 to 5—1 being very dissatisfied and 5 being very satisfied. This would create an ordinal variable because the responses are ordered from least to most satisfied. When dealing with an ordinal variable, it is important to remember that the difference between levels is not necessarily equal; for example, the difference between “satisfied” and “very satisfied” may be greater than the difference between “dissatisfied” and “slightly dissatisfied”.
Characteristics of Ordinal Variables
Ordinal variables are variables that are used to measure qualitative traits and establish a relative rank. They do not have a standardized interval scale and therefore cnnot be used to perform mathematical operations. However, the median and mode of ordinal variables can be analyzed. Additionally, ordinal variables often involve assigning numbers or labels to categories so that they can be put in order. For example, if we were rating someone’s attitude on a scale of 1-5, the numbers would represent different categories (e.g., 1=very bad attitude; 5=very good attitude).
Identifying an Ordinal Variable
If a variable is ordinal, it will be listed in an ordered manner, usualy with numbers indicating the order of the list. The numbers are not mathematically measured or determined, but assigned as labels for opinions. For example, numerical ratings (1-5) on a survey are often used to measure ordinal data, where 1 is the lowest rating and 5 is the highest rating. Additionally, if the categories cannot be meaningfully quantified into numbers or compared mathematically, then it is likely that the data is ordinal.
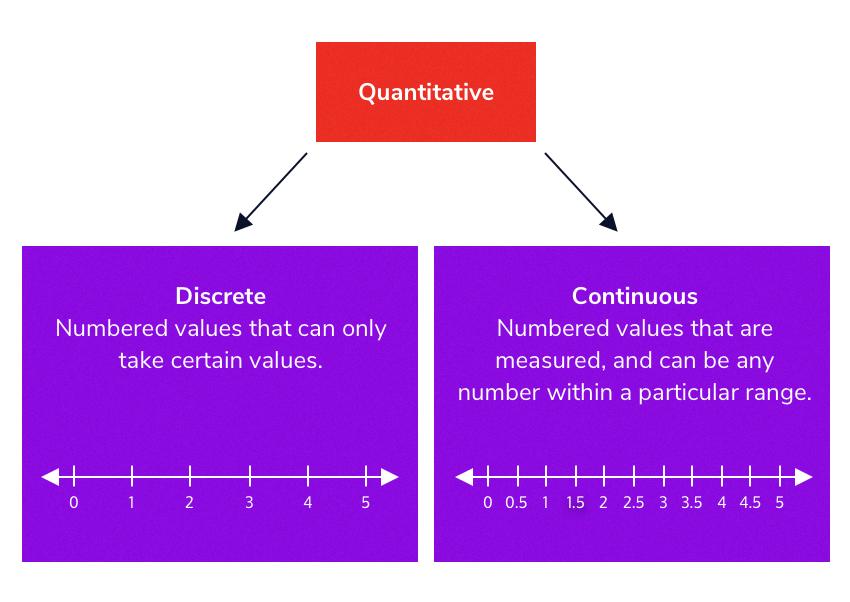
Types of Variables: Discrete vs. Continuous
Time is not a continuous variable. Time is usally measured in discrete units, such as seconds, minutes, hours, days, etc. Therefore, time is not a continuous variable since it can only take on specific values and not infinitely many values between the maximum and minimum.
Difference Between Ordinal and Categorical Variables
Ordinal variables differ from categorical variables in that they imply an ordering among the categories. In oher words, each category has a particular rank or position within the set of categories. This rank or position is what distinguishes an ordinal variable from a categorical variable. Unlike categorical variables, which do not necessarily have any inherent order, ordinal variables can be ranked and compared to each other. For example, in our economic status example, low would be ranked as lower than medium and high would be ranked as higher than medium. This makes it possible to measure how far apart two categories are from one another, allowing for further analysis and comparison among categories.
Types of Education Measurement
Education is an ordinal variable, meaning that it is measured on a scale with different levels of order. Education level can be categorized into three distinct groups: high school, undergraduate degree, and graduate degree. Each of these categories has a higher vlue than the preceding one, which means that education level can be arranged in an ordered way. This makes education an ordinal variable rather than a continuous one.
Can an Ordinal Likert Scale Be Considered a Continuous Variable?
Yes, an ordinal Likert scale can be treated as a continuous variable. This is becuse Likert scales have five or more categories, which allows them to be used in continuous analysis. This has been supported by research in the field, with studies demonstrating that treating Likert scales as continuous variables does not adversely affect the results (Johnson & Creech, 1983; Norman, 2010; Sullivan & Artino, 2013; Zumbo & Zimmerman, 1993). When using such scales as a continuous variable it is important to remember that the categories are still separate and cannot be averaged together.
Identifying Continuous and Quantitative Variables
Continuous quantitative variables are those which can take any values wthin a range. Examples of continuous quantitative variables include body mass, height, blood pressure, cholesterol, temperature, time, speed and distance. These variables are measured on a continuous scale and can take any value within the given range. For example, body mass can be measured in kilograms or pounds, height in centimetres or inches, temperature in Celsius or Fahrenheit and so on. Continuous quantitative variables can also be expressed as percentages or fractions.
Continuous and Discrete Variables
Discrete variables are those that take on distinct, countable values. Examples of discrete variables include items such as age (which coud be counted in years), the number of children in a family, or the number of cars owned. Discrete variables can only take on certain values—there is no possibility for fractional or decimal values.
Continuous variables are those that take on any value within a range, and the number of possible values within that range is infinite. Examples of continuous variables include items such as temperature (which could be measured in degrees Celsius or Fahrenheit), height (measured in inches or centimeters), or time (measured in seconds). Continuous variables can take on any value within a certain range—including fractional and decimal values.
Conclusion
In conclusion, ordinal variables are not necessarily continuous. While they can sometimes be numerical, they cannot be treated as such and certain mathematical operations cannot be performed on them. Ordinal variables are considered categorical variables and are distinct from continuous variables which can be either ordinal, interval, or neither.
