Momentum is a physical quantity that is essential in the study of mechanics. It has been a topic of discussion and confusion for many students and even professionals in the field of physics. Understanding the nature of momentum is crucial in comprehending the behavior of moving objects and the principles that govern their motion.
One of the fundamental questions that arise when discussing momentum is whether it is a scalar or vector quantity. To answer this, we need to understand the basic concepts of scalar and vector quantities.
A scalar quantity is a physical quantity that only has magnitude, which means that it can be described by a single value or number. Examples of scalar quantities include distance, speed, temperature, and mass. In contrast, a vector quantity has both magnitude and direction. Examples of vector quantities include displacement, velocity, acceleration, and force.
Momentum is defined as the product of mass and velocity. Since mass is a scalar quantity and velocity is a vector quantity, we can derive that momentum must, therefore, be a vector quantity. This means that momentum has both magnitude and direction.
To understand how momentum can have direction, let’s consider a simple example. Suppose we have two objects with the same mass, but one is moving to the right with a velocity of 5 m/s, and the other is moving to the left with a velocity of 5 m/s. Although both objects have the same mass and speed, they have different momenta since they are moving in opposite directions. Therefore, the direction of the momentum is determined by the direction of the velocity.
Another way to understand the vector nature of momentum is to consider the principle of conservation of momentum. According to this principle, the total momentum of a system of objects is conserved if thee are no external forces acting on the system. This means that the total momentum of the system before a collision is equal to the total momentum after the collision. However, this principle only holds true if we consider the momentum as a vector quantity, taking into account both magnitude and direction.
Momentum is a vector quantity since it has both magnitude and direction. This means that understanding the direction of momentum is crucial in understanding the behavior of moving objects and the principles that govern their motion.
Why Is Momentum Scalar?
Momentum is not a scalar quantity, rather it is a vector quantity. The reason behind it is that momentum is the product of the mass and velocity of an object, where mass is a scalar quantity and velocity is a vector quantity. Since momentum is the product of a scalar and a vector, it becomes a vector quantity. It has both magnitude and direction, and its direction is the same as the direction of velocity. Thus, it is incorrect to say that momentum is a scalar quantity.
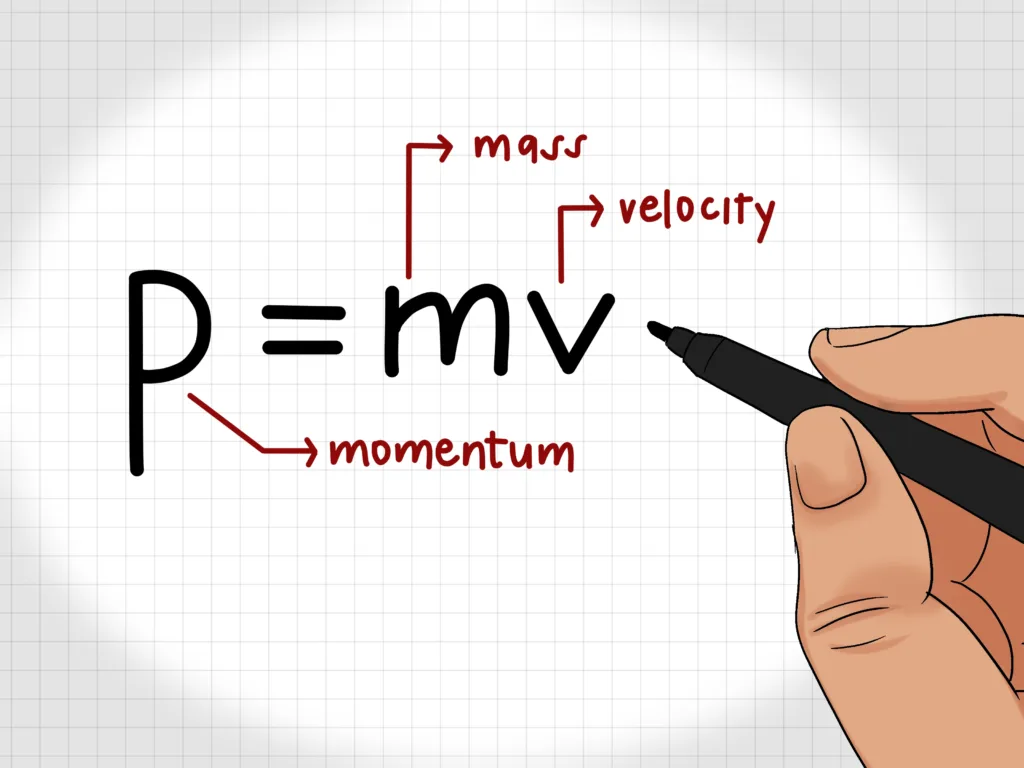
Why Momentum Is Not Scalar Quantity?
Momentum is not a scalar quantity because it is a product of two vector quantities – mass and velocity. Mass is a scalar quantity as it only has magnitude and no direction. Velocity, on the other hand, is a vector quantity as it has both magnitude and direction. When we multiply a scalar quantity with a vector quantity, the result is a vector. Thus, the product of mass and velocity, which is momentum, is also a vector quantity.
To further explain, let’s consider an example. Suppose we have two objects with the same mass but different velocities. Object A has a velocity of 5 m/s to the right, while object B has a velocity of 5 m/s to the left. Although both objects have the same mass, they have different momenta due to ther opposite directions of motion. Object A has a momentum of 25 kg m/s to the right, while object B has a momentum of 25 kg m/s to the left. If momentum were a scalar quantity, both objects would have the same momentum, which is not the case.
Momentum is a vector quantity because it is a product of two vector quantities – mass and velocity. Scalar quantities only have magnitude, while vector quantities have both magnitude and direction.
Why Is Momentum Considered A Vector?
Momentum is considered a vector because it has both magnitude and direction. This means that it not only has a numerical value, but also a specific orientation in space. In physics, a vector is defined as a quantity that has both magnitude and direction, and momentum fits this description perfectly.
To understand why momentum is a vector, it’s important to consider its formula: p = mv. Here, p represents momentum, m represents mass, and v represents velocity. As you can see, both mass and velocity are included in the formula, which are both vector quantities. Mass has a magnitude and direction in the form of its position in space, and velocity has both magnitude and direction because it is a vector quantity.
When you multiply mass and velocity togther to calculate momentum, the resulting quantity also has a magnitude and direction. The magnitude of momentum is determined by the mass and velocity of the object, while the direction of momentum is determined by the direction of the velocity vector. This is why momentum is considered a vector quantity in physics.
Momentum is considered a vector because it has both magnitude and direction. It is a product of mass and velocity, which are both vector quantities, and as a result, it also has a specific orientation in space.
Conclusion
Momentum is not a scalar quantity, but rather a vector quantity. This is due to the fact that momentum is a product of the mass of an object, which is a scalar quantity, and its velocity, which is a vector quantity. As such, momentum possesses both magnitude and direction, making it a vector quantity. It is important to remember that understanding the nature of momentum is crucial in various fields of study, especially in physics and engineering, whee it is used to describe the behavior of moving objects. Therefore, it is essential to have a clear understanding of the concept of momentum and its properties to accurately analyze and predict the motion of objects.
