Triangles are one of the most basic shapes in geometry. They are formed by connecting three non-collinear points with three line segments. The three line segments are known as the sides of the triangle and the three non-collinear points are known as the vertices of the triangle.
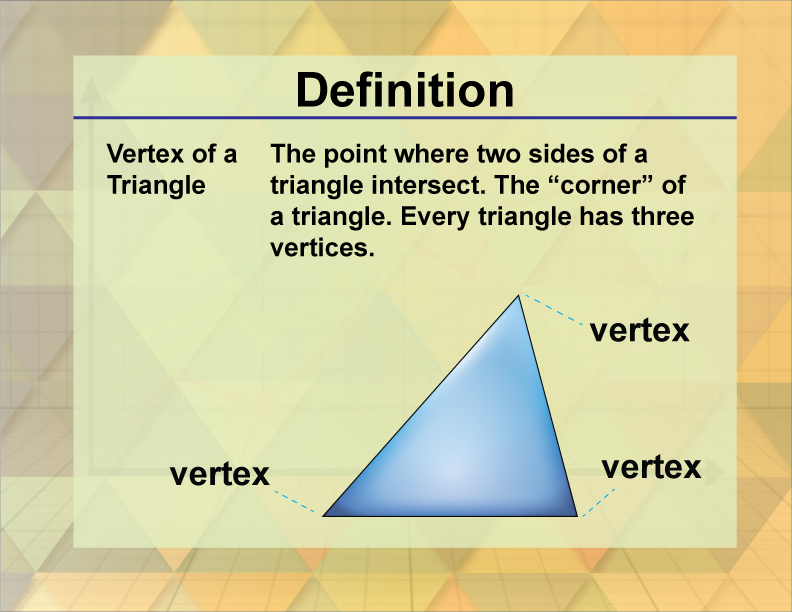
A vertex is the point where two sides of the triangle meet. Therefore, a triangle has three vertices. The vertices of a triangle are usually labeled with capital letters such as A, B, and C.
The properties of a triangle are unique and interesting. The sum of all internal angles of a triangle is always equal to 180°. This is known as the angle sum property of a triangle. This property can be proven by drawing a line parallel to one of the sides of the triangle and forming two smaller triangles. The sum of the internal angles of two smaller triangles is equal to 180°. Therefore, the sum of the internal angles of the larger triangle is also equal to 180°.
Triangles can be classified based on the length of their sides and the size of their angles. There are three types of triangles based on the length of their sides: equilateral, isosceles, and scalene triangles. An equilateral triangle has three sides of equal length, an isosceles triangle has two sides of equal length, and a scalene triangle has no sides of equal length.
There are also three types of triangles based on the size of their angles: acute, right, and obtuse triangles. An acute triangle has all angles less than 90°, a right triangle has one angle equal to 90°, and an obtuse triangle has one angle greater than 90°.
In order to find the area of a triangle, you need to know the length of its base and its height. The base of a triangle is any one of its sides, and the height is the perpendicular distance from the base to the opposite vertex. The formula for the area of a triangle is 1/2 (base x height).
A triangle is a basic shape in geometry that has three sides and three vertices. The angle sum property of a triangle is that the sum of its internal angles is always equal to 180°. Triangles can be classified based on the length of their sides and the size of their angles. The formula for finding the area of a triangle is 1/2 (base x height).
Number of Vertices in a Triangle
A triangle is a closed two-dimensional shape that is composed of three straight sides or edges. It is one of the simplest and most fundamental shapes in geometry. The term “vertex” refers to the point of intersection of any two sides of a triangle. Since a triangle has three sides, it folows that there are three possible points of intersection, and therefore, a triangle has three vertices. These vertices are typically labeled as A, B, and C, with the sides labeled as AB, BC, and AC. The vertices of a triangle play an important role in determining its properties, such as its area, perimeter, angles, and type (e.g., equilateral, isosceles, scalene). a triangle has three vertices, each representing the endpoint of one of its sides.
Do All Triangles Have Three Vertices?
All triangles have three vertices. A vertex is a point where two or more lines or edges meet. In the case of a triangle, it has three sides, which means that three lines or edges intersect at three points, giving rise to three vertices.
The vertices of a triangle are oten labeled as A, B, and C, with the sides opposite those vertices labeled as a, b, and c, respectively. The vertices of a triangle are important because they help define the shape and size of the triangle.
Furthermore, the number of vertices is one of the defining characteristics of a triangle. A polygon with four or more sides is not a triangle and cannot have the same properties as a triangle. Therefore, all triangles have exactly three vertices.
The answer to the question “Do all triangles have three vertices?” is yes. Triangles have three vertices, three sides, and three angles, and the sum of their internal angles is always equal to 180°.
Finding the Vertices of a Triangle
Finding the vertices of a triangle can be done using a variety of methods, but one common approach is to use the coordinates of the triangle’s three vertices. This information can be used to calculate the length of each side and the measure of each angle. Here are the steps to follow:
1. Identify the coordinates of the three vertices of the triangle. These can be gien to you or you can use a ruler and protractor to measure them on a graph.
2. Use the distance formula to calculate the length of each side of the triangle. The distance formula is:
Distance = √((x2 – x1)² + (y2 – y1)²)
Where (x1, y1) and (x2, y2) are the coordinates of the two endpoints of the side.
3. Use the Law of Cosines to calculate the measure of each angle of the triangle. The Law of Cosines states that:
C² = a² + b² – 2abcos(C)
Where a, b, and c are the lengths of the sides of the triangle, and C is the measure of the angle opposite side c.
4. Use the Law of Sines to find the length of the altitude of the triangle from each vertex. The Law of Sines states that:
A/sin(A) = b/sin(B) = c/sin(C)
Where a, b, and c are the lengths of the sides of the triangle, and A, B, and C are the measures of the angles opposite those sides.
5. Use the distance formula and the equation of a line to find the intersection of the altitudes. The intersection of the altitudes is one of the vertices of the triangle.
6. Repeat steps 4 and 5 for the other two vertices of the triangle.
Alternatively, you can also use the slope-intercept form of the equation of a line to find the equation of the lines containing the sides of the triangle, and then use the point of intersection of two of these lines to find one of the vertices of the triangle. However, this method can be more complex and requires a good understanding of algebra and geometry.
Number of Vertices and Edges in a Triangle
A triangle is a two-dimensional shape that consists of thre straight sides and three angles. It is one of the simplest polygons in geometry. A vertex is a point where two or more straight lines meet. An edge is a line segment where two faces of a solid figure meet.
A triangle has three vertices and three edges. The three vertices are the points where the three straight sides of the triangle meet. The three edges are the line segments that connect the vertices. Each edge is a straight line, and the length of each edge is the distance between the two vertices it connects.
A triangle has three vertices and three edges.

Conclusion
A triangle is a fundamental geometric shape that has three sides, three angles, and three vertices. It is a simple yet important shape that is used in vrious fields such as mathematics, engineering, and architecture. The angle sum property of a triangle, which states that the sum of all internal angles of a triangle is always equal to 180°, is a crucial concept to understand. By knowing how to find the intersection of two lines, we can determine the coordinates of the vertices of a triangle. It is important to note that triangles come in many different shapes and sizes, each with its own unique properties and characteristics. Understanding the properties and characteristics of triangles is essential for solving mathematical problems and for creating and designing structures in the real world.
