Standard deviation is an important measure of how much variation there is in a set of data. It is calculated by taking the square root of the variance, which is the average of the squared differences from the mean. In other words, it measures how far individual values in a dataset are from the mean, and can be used to help identify outliers or trends in data.
When dealing with standard deviation, it’s important to remember that it can never be negative due to its definition. The standard deviation measures a distance (either way) from the mean, and so its result can never be negative. It is always positive because any negative value would indicate that a value was closer to the mean than 0 – which isn’t possible by definition.
That said, while standard deviation cannot be negative, individual numbers that you calculate standard deviation for can reach any value, including negative. This means that while individual values may have a negative impact on standard deviation itslf (by increasing it), they cannot cause it to become negative.
In conclusion – while individual values may be negative and have an impact on standard deviation, standard deviation itself will always remain positive due to its definition as a measure of distance from the mean.
Is Standard Deviation Always Positive?
No, the standard deviation is not only positive. While it is true that the convention for measuring the standard deviation is to take the absolute value of each data point’s distance from the mean (so as to avoid any negative values), this does not mean that the standard deviation can only be positive. In fact, if all of your data points have a negative distance from the mean, then you will end up with a negative standard deviation.
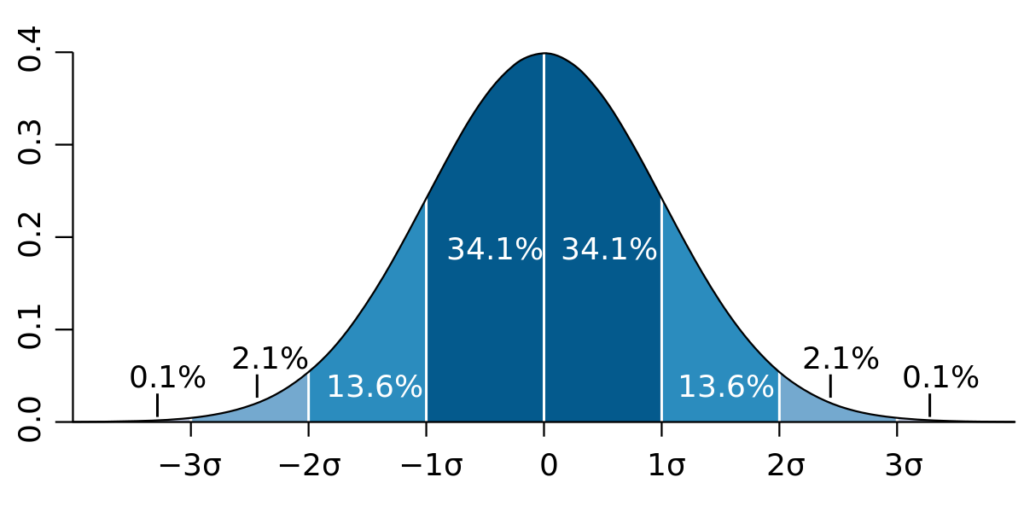
Finding Negative Standard Deviation
It is not possible to find a negative standard deviation. Standard deviation is a measure of how spread out a set of data points are from the mean, and it must always be equal to or greater than zero. Negative values would indicate that the data points are closer to the mean than they actualy are, which would provide an inaccurate measure of the variability in the data set.
However, it is possible for individual items in your data set to have negative values. When calculating standard deviation, these negative values will contribute to the overall result just as positive values do.
Negative Standard Deviation: What Does It Mean?
If you calculate a negative standard deviation, it means that there is an error in the data set or in the formula used to calculate it. A negative standard deviation cannot exist in reality, as it implies that the data points are farther away from each other than the mean, which is mathematically impossible. To fix this issue, you should go back and double-check your data set and/or the formula used to calculate standard deviation.
Can Standard Deviation Be Less Than One?
Yes, standard deviation can be less than 1. Standard deviation measures the spread of data from its mean or average value, and it is expressed in the same unit of measurement as the data itself. A standard deviation of less than 1 indicates that the data points are clustered very closely around the mean and that there is not much variation among them. For example, if a group of students had test scores that all fall within one point of the average score, then their standard deviation would be less than 1.
Can Negative Values Exist for Variance and Standard Deviation?
No, variance and standard deviation (SD) cannot be negative. Variance is a measure of how spread out numbers are in a given set of data, while the standard deviation is the square root of the variance. Mathematically, the variance of any set of data can never be negative; it can only be zero or greater. In a situation where all values in a data set are equal, then the variance would be equal to zero. This would also mean that the standard deviation would also be equal to zero as its value is derived from the variance.
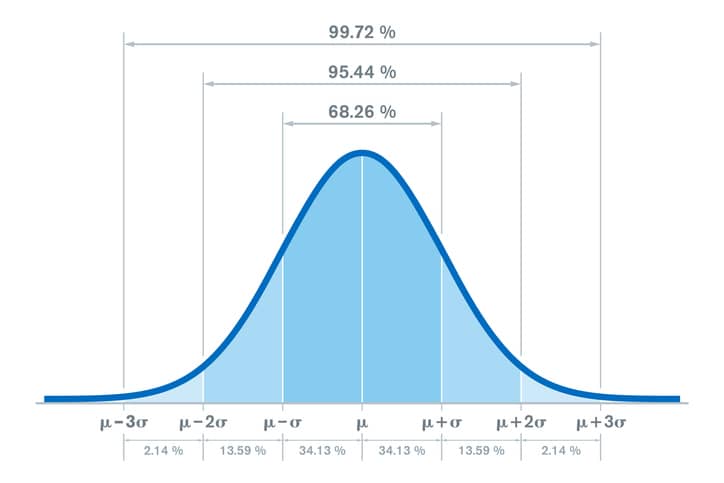
Can Standard Deviation be Equal to Zero?
Yes, the standard deviation can be zero. This happens when all of the values in a sample are identical. In this case, there is no variability in the sample, and so the standard deviation is equal to zero.
Distribution with Negative Standard Deviation
It is not possible for a distribution to have a negative standard deviation. The standard deviation is a measure of the spread or variability of values within a dataset and is always calculated using the square root of a number, which cannot be negative. Therefore, there is no type of data distribution with a negative standard deviation.
Conclusion
The standard deviation is a powerful tool for understanding the spread of data within a set. It measures the amount of variability or dispersion from the average or mean of the set. By convention, it is always expressed as a positive number, as it measures distance from the mean in both directions. This metric can be used to compare different sets of data and to identify outliers that may exist within a set. Standard deviation provides valuable insight into how closely related various values are within a dataset.
