Are all isosceles triangles similar? It’s a question that can ofen cause confusion! An isosceles triangle is a triangle with two equal sides and two equal angles opposite the equal sides. While all isosceles triangles have the same basic shape, they may not all be similar in size. To understand why, it helps to take a closer look at the properties of isosceles triangles.
In an isosceles triangle, if two sides are equal, then the angles opposite to the two sides correspond to each other and are always also equal. This means that if two isosceles triangles have the same angles opposite their two equal sides, then they will be similar in size. However, if they have different angles opposite their two equal sides, then they will not be similar in size.
It’s also important to note that for any triangle (not just an isosceles triangle), similarity does not depend on side lengths alone; it must also take into consideration angles. For any set of three sides of a triangle to be similar, the ratio between those three sides must be constant across each set of triangles beig compared.
For example, with equilateral triangles, each side length is equal and therefore the ratio between any two diferent side lengths will remain constant across all equilateral triangles; thus making them all similar in size.
So while all isosceles triangles have the same basic shape and can sometimes be similar in size as well; it ultimately depends on whether or not their corresponding angles match up as well.
Are Isosceles Triangles Always Similar?
No, not all isosceles triangles are always similar. While two isosceles triangles may be congruent to one another, which would make them similar, the similarity of two isosceles triangles depends on other factors as well. For example, two isosceles-right triangles are similar if their correspoding angles are congruent; however, for any other type of isosceles triangle this does not necessarily mean that they are similar. Additionally, the diagonals of a trapezium do not divide each other into proportional segments, meaning that even if two shapes have the same number of sides and angles, they may still not be similar.
Source: homeschoolmath.net
Are All Equilateral Triangles Similar?
Yes, all equilateral triangles are similar. This is because, in an equilateral triangle, all three sides are equal in length. Therefore, the ratio between two sides of any two equilateral triangles will be constant, making them similar. Additionally, all interior angles of an equilateral triangle are equal to 60 degrees, again making them similar.
Are Isosceles Triangles Congruent?
No, all isosceles triangles are not congruent. Congruent triangles are defined as having the same exact size and shape, meaning that two isosceles triangles can be congruent if they have the same length of sides and the same angle measurements. However, many isosceles triangles have different side lengths or angle measurements, making them not congruent.
Are All Isosceles Angles Equal?
No, not all isosceles angles are the same. In an isosceles triangle, two of the sides are equal and the angles opposite to those two sides are also equal. However, the third angle may be different from the other two.
Properties of Isosceles Triangles
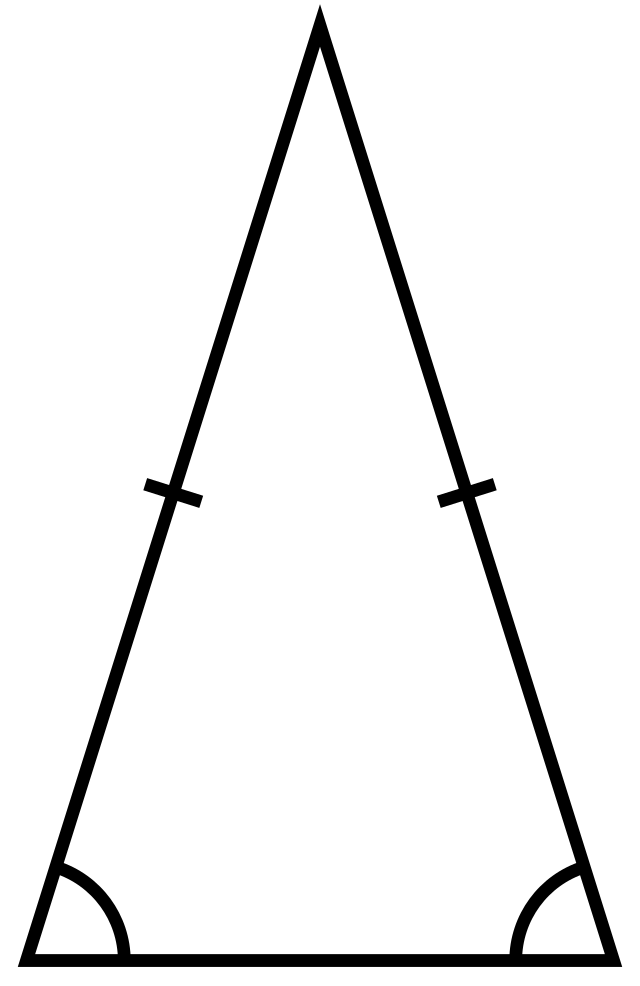
All isosceles triangles have two sides that are equal in length, with the tird side being the base. The two angles opposite to the equal sides are congruent to each other and the triangle has an overall symmetry due to the two equal sides. The angle between the two equal sides and the base of an isosceles triangle is always less than 90 degrees. This angle, known as the vertex angle, is always less than or equal to the other two angles. In addition, all isosceles triangles have a point of reflectional symmetry along its axis, which means that if you were to draw an imaginary line down its center, both sides would be mirror images of each other.
Similarity of Triangles
Equilateral triangles are alays similar. An equilateral triangle is a triangle with three equal sides and three equal angles, making all angles 60 degrees. Since all sides and angles of an equilateral triangle are congruent, two equilateral triangles will always have the same shape and size, meaning they are similar.
Are All Scalene Triangles Similar?
No, all scalene triangles are not similar. Scalene triangles are defined as having three sides of dfferent lengths and three angles of different measures. This means that there can be a wide variety of scalene triangles, meaning they can have different shapes and sizes which would make them not similar to each other.
Are All Triangles Similar?
No, not all triangles are similar either equilateral or isosceles. Triangles can be categorized into various types based on the length of their sides and the angles between them. Equilateral triangles have thee sides of equal length, and all three angles are also equal in measure. Isosceles triangles also have two equal length sides, but the third side and its angle may be different from the other two sides. Other triangle types include scalene, right, obtuse and acute triangles. Each type has unique characteristics that distinguish it from the others.
Are All Right Triangles Similar?
No, not all right triangles are similar. For two triangles to be similar, the ratios between the corresponding sides of each triangle must be equal. However, this does not necessarily hold true for right triangles, as there can be two right triangles with different side lengths and different ratios between their corresponding sides. As such, two right triangles can have the same angles but still have different side lengths and therefore not be similar.
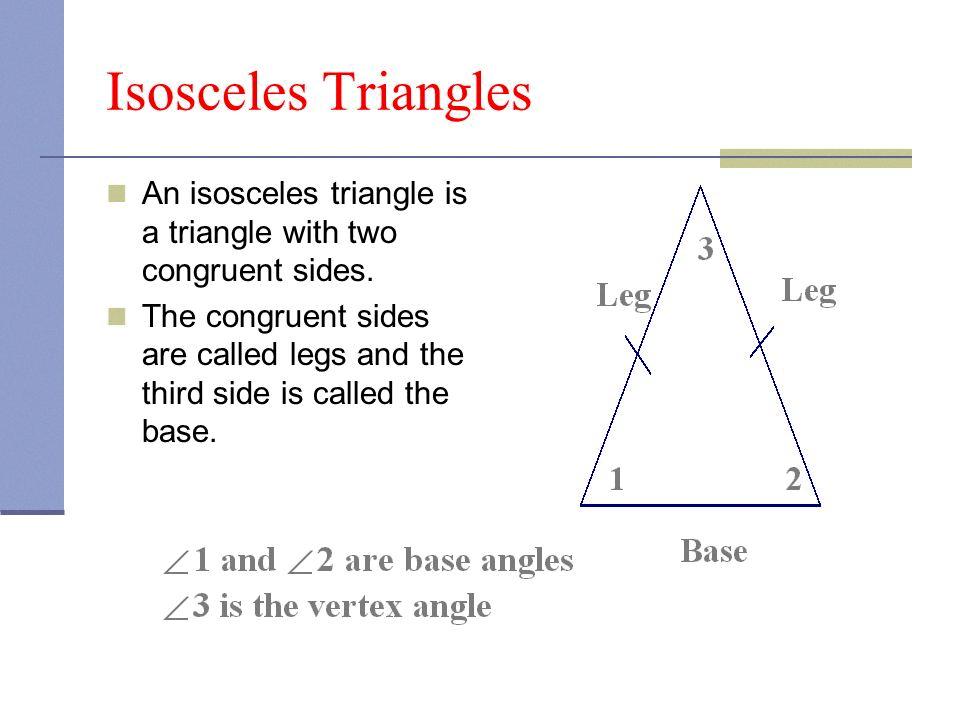
Properties of an Isosceles Triangle
An Isosceles Triangle has five distinct properties:
1. It has two sides of equal length.
2. The angles opposite to equal sides are equal in measure.
3. The altitude from vertex A to the base BC is the perpendicular bisector of the base BC.
4. The altitude from vertex A to the base BC is the angle bisector of the vertex angle ? A.
5. The line segment connecting the midpoints of two sides is parallel to and half as long as the third side of the triangle.
The Symmetry of Isosceles Triangles
No, an isosceles triangle does not have to be symmetrical. An isosceles triangle has two equal sides, but the angles of the triangle may not be equal. If the angles are unequal, then the triangle will not have any lines of symmetry and therfore will not be symmetrical.
Congruence of an Isosceles Triangle
An isosceles triangle is congruent when its sides and angles match exactly the same measurements. This means that the two legs of the triangle must be equal in length, and the two base angles opposite of these legs must also be equal in measurement. Additionally, the vertex angle formed by the two legs that join together must also match in measure. When all three of these elements—legs, vertex angle, and base angles—have identical measurements to another isosceles triangle, then they are considered congruent.
Are Acute Triangles Similar?
No, not all acute triangles are similar. Two acute triangles can be similar if the corresponding angles are equal. This is known as the angle-angle (AA) postulate. However, two acute triangles can have unequal corresponding angles and still be considered congruent if the other corresponding sides and angles are equal. This is known as the side-angle-side (SAS) postulate. Therefore, it is possibe for two acute triangles to be either similar or congruent even though they are both acute triangles.
Source: slideplayer.com
Similarity of Isosceles Trapezoids
No, not all isosceles trapezoids are similar. While they do share certain properties such as two parallel sides of equal length (the bases), two other sides (the legs) of equal length, and two diagonals of equal length, the size of each side can vary greatly from one isosceles trapezoid to another. Therefore, even though they share the same properties, they will have different shapes and sizes and are not necessarily similar.
Conclusion
In conclusion, isosceles triangles are a special type of triangle that have two sides of equal length and two angles of equal measure. They are also similar to any other isosceles triangle regardless of size. This makes it easy to identify the relationships between the sides and angles in an isosceles triangle. Furthermore, it is important to remember that not all isosceles right triangles are congruent as they may differ in size. As such, it is essential to take into account all properties of an isosceles triangle before making conclusions about its similarity or congruence with other shapes.
